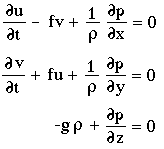
The analysis starts from the linearised Equations of Motion for a frictionless ocean:
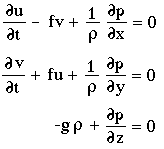
The third equation is also known as the hydrostatic equation.
If the equations are applied to a narrow basin or gulf, movement can only occur in two dimensions and the Coriolis force can be neglected. Taking the axis of the gulf in the x-direction we then have:
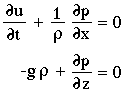
The stratification can be ignored in the investigation of surface tides, so density ρ can be replaced by a constant reference density ρ0, and the hydrostatic equation can be integrated to give pressure p as
p = g (H + ζ)
where H is the depth and ζ(x) the surface elevation, or the shape of the sea surface along the basin. This expression for p can be introduced into the Equation of motion. For a basin of uniform (constant) depth this leads to
When this result is multiplied with the (constant) width B of the basin the result is
Where S = B . H is the cross-sectional area and q = u . B is the transport through the cross-sectional area.
We now have an equation for the unknown target variable ζ(x) and the unknown transport q(x), which we have to eliminate. This is done with the help of the continuity equation, which for an ocean of constant density is
When vertically integrated and multiplied by the width of the basin this equation becomes
| continue with model equations |
back to co-oscillation tides |
This utility is based on A. K. Easton (1978) A reappraisal of the tides in Spencer Gulf, South Australia. Australian Journal of Marine and Freshwater Research 29, 467 - 477.
Last updated 23 August 2000. Contact address: